Nonlinear Dynamics in Optical Systems The group's research activity in the field of nonlinear dynamics and chaos in optics is a forefronting effort in the UK and is well respected internationally for its many contributions which span some 20 years. Chaos and Control in Lasers and Nonlinear Devices Chaos is an inherent feature of many nonlinear systems. In particular, the transition from order to disorder occurs with universality, irrespective of physical properties of the systems. Chaos occurs in optics, both in lasers and in nonlinear optical devices. Such systems, which are fundamentally simple both in construction and in the mathematics that describe them, provide excellent opportunities for investigating these nonlinear phenomena as well as for technological innovation. Our experimental and theoretical work addresses chaos and control of chaos. Through the new perspectives of nonlinear dynamical theory these programmes explore the universality of such behaviour, its physical origins and the bearing it has on conventional understanding of nonlinear optical interaction phenomena. Among these are nonlinear mixing and coupling, phase conjugation and nonlinear beam propagation. The systems researched include solid-state lasers, gas lasers, optical fibres and optical devices such as nonlinear switching systems and phase conjugations. Recent contributors have led to the discoveries of instabilities and chaos in beam propagation in optical fibre through stimulated scattering and nonlinear refraction and classifiable routes to chaos in solid state and gas laser systems. Figure: Time evolution of transverse field distribution of a laser showing turbulent emission (a) and its evolution (b) and (c) to a striped pattern (d) when feedback control is applied Pattern Formation in Optical Systems Pattern formation and spatiotemporal complexity is an important branch in the field of nonlinear dynamics and chaos. It concerns the tension between order and randomness through which patterns spontaneously emerge and disappear into turbulence. Spatiotemporal chaos (turbulence) occurs when different types of motion, excited in local regions in an extended system, interact to destroy the spatial coherence of the system concurrent with the onset of temporal chaos. In optics this arises from local nonlinearity and diffractive coupling in spatially extended systems such as broad area devices and lasers. This phenomenon in continuous physical systems is described by partial differential equations. Our investigations are by means of computational simulations augmented by theory through stability and bifurcation analysis and symmetry consideration. Recent contributions include numerical observations of different pattern formations, in particular spiral waves and turbulent waves, in lasers, analysis of optical spiral-forming geometry, and explanation of their physical origin in terms of nonlinear optical interactions. Current research interests of the group are in the areas of optical excitability and associated pattern formations, both in lasers and nonlinear optical devices. Excitability underlies a class of pattern forming phenomena normally associated with chemical and biological systems. Our research into this subject explores the universality of this phenomenon and its potential applications in modern optical technology. 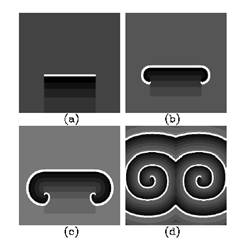Figure: Transverse field of laser emission from a broad area laser showing spiral wave pattern These theoretical research programmes have strong interaction with the experimental research activities in the Department. Our combined theoretical and experimental effort is in the areas of control of chaos and spatiotemporal chaos in optical devices. Here the theoretical work has resulted in the development of new algorithms for controlling patterns in both low-dimension and high-dimensional dynamical systems, some of which have been successfully adopted in experiments.